728x90
반응형
💡순열(Permutation)
: 서로 다른 n개의 원소에서 r개를 중복없이 순서에 상관있게 선택하는 혹은 나열하는 것을
순열(permutation)이라고 한다. (나무위키)

//nPr
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
N = sc.nextInt();
R = sc.nextInt();
input = new int[N];
numbers = new int[R];
isSelected = new boolean[N]; // 방문 여부를 체크하기 위함
for (int i = 0; i < N; i++) {
input[i] = sc.nextInt();
}
permutation(0);
}
private static void permutation(int cnt) {
if(cnt == R) { // R개의 원소를 다 뽑았다면 return
System.out.println(Arrays.toString(numbers));
return;
}
for(int i=0; i<N; ++i) {
//뽑으려는 수가 사용 됐다면
if(isSelected[i]) continue;
// 뽑으려는 수가 사용되지 않았다면
// 배열에 넣어줌
numbers[cnt] = input[i];
// 사용처리 true
isSelected[i] = true;
permutation(cnt+1);
// R개의 원소 생성 후 사용처리 다시 해제
isSelected[i] = false;
}
}
🚨 주요 포인트
boolean타입의 isSelected 배열을 통해서 원소를 뽑았는지 안뽑았는지 체크하는 것이 포인트다
중복 순열의 경우(1,1,1), (1,2,1),(2,1,1)isSelected 배열을 통해 원소 체크를 하지 않아도 된다
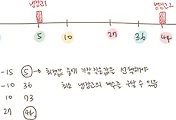
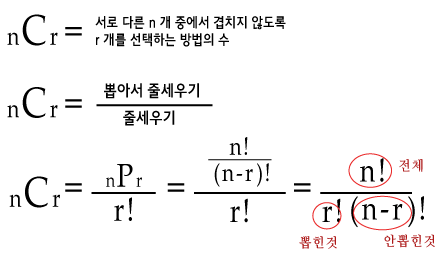
💡조합(Combination)
: 서로 다른 n개의 원소를 가지는 어떤 집합 (사실, 집합은 서로 다른 원소의 모임으로 정의된다.)
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
N = sc.nextInt();
R = sc.nextInt();
input = new int[N];
numbers = new int[R];
for (int i = 0; i < N; i++) {
input[i] = sc.nextInt();
}
combination(0,0);
}
private static void combination(int cnt,int start) {
if(cnt == R) { //R개의 원소를 다 뽑았다면
System.out.println(Arrays.toString(numbers));
return;
}
for(int i=start; i<N; i++) { //i =start
// 배열에 넣어줌
numbers[cnt] = input[i];
// 다재귀를 start+1이 값이 아닌 i+1
combination(cnt+1,i+1);
}
}
🚨 주요 포인트
조합은 start를 기준으로 (현재 뽑힌 수 다음 i+1)를 호출하기에 중복될 일이 없고 (2,4,6) (4,2,6)과 같은 경우도 제거한다.
중복 조합의 경우, 재귀로 넘길 때 start의 값을 신경 써주면 된다combination(cnt+1, i) // 자기 자신도 포함될 수 있게
💡부분집합(SubSet)
: 집합 A={a, b, c}의 모든 부분집합을 찾아 나열하면 다음과 같다.
∅, {a}, {b}, {c}, {a,b}, {b,c}, {a,c}, {a,b,c} 즉, A의 부분집합의 총수는 2^n개이다
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
N = sc.nextInt();
input = new int[N];
isSelected = new boolean[N];
for(int i=0; i<N; ++i) {
input[i] = sc.nextInt();
}
generateSubSet(0);
}
//count: 현재까지 고려한 원소 수
private static void generateSubSet(int count) {
if(count==N) {
for(int i=0; i<N; ++i) {
if (isSelected[i]){
System.out.print(input[i]+" ");
}
}
System.out.println();
return;
}
// 원소 선택
isSelected[count] = true;
generateSubSet(count+1);
// 원소 미선택
isSelected[count] = false;
generateSubSet(count+1);
}
🚨 주요 포인트
원소를 선택하는 것과 그렇지 않을 때,
2가지로 나누어 구현하는 것이 중요
728x90
'Algorithm' 카테고리의 다른 글
| [정올] 1828: 냉장고(Java) (0) | 2022.08.30 |
|---|---|
| [BJ] 1541번: 잃어버린 괄호(Java) (0) | 2022.08.30 |
| [Java] 조합 (Combination) (0) | 2022.08.30 |
| [Java] 순열(permutation) (0) | 2022.08.30 |
| [Java] BFS(Breadth First Search) (0) | 2022.08.30 |